Music Composer, music recognition software. Composer recognizes polyphonic music from a microphone or other Wave input or file and converts it into Midi sequences. You can sing, whistle or play guitar, piano, flute and so on with your microphone, Composer will automatically recognize and score your music and create standard Midi sequences. No Midi keyboard or musical experience required.
AKoff Guitar Assistant designed to assist in visual tuning a guitar with microphone or an electrical guitar connected to PCs sound card. It analyzes in real time a stream of audio signals from WAVE input of your sound card and calculates the main frequency. This frequency is shown by a pointer on graphic guitar signature stamp (frets), comparing it to the proper frequency. Tuning a string now simply means centering the pointer on the appropriate graphic fret.
Why is math so fascinating? At least since the time of the ancient Babylonians, people have found mathematical concepts absolutely wonderous. Among the many wonders is how a human game, mathematics, can correlate so well with the workings of the real physical world. Beyond that unanswerable question is the question of how much math can tell us. What are the limits of knowledge of the physical world based on mathematics.
Goedel, Einstein, Newton, Liebniz, Descarte, Euclid and hundreds more gave us the rich and fascinating world of mathematics. This webpage is devoted to sharing the wonder!
|
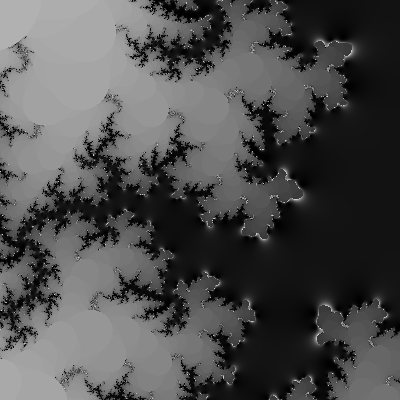
The Mandelbrot Set is that beautiful fractal made famous in the 1980's with wonderful photographs that brought complex dynamics to popular culture. Or rather, brought the subject of complex dynamics into the discussions in the coffee shops and made mathematics just a little cooler in the eyes of the young. |
| Images of the Mandelbrot set created by a simple algorithm and rendered by a simple matlab application are shown and the method of creating these images is described. |
|
Gödel's incompleteness theorems put a limit on what a system of mathematics can claim about itself. They may also show a limit for what we can know. |
Platonic Solids, more exciting than a platonic relationship. A discussion of symmetry, regular polygons, platonic solids, and the Euler Characteristic
|
The Sobel operator is a commonly used image processing method for finding edges in an image. You can use it to quickly find outlines of objects in a photo or video feed. |
| If you have ever wondered how to easily get an outline from an image, such as a photograph or even a live camera feed, this article describes a simple process using the Sobel operator. With this method you can take a photo and quickly draw just the outlines of the objects in the photo. This is a commonly used image processing operation that allows machines to find information that your brain automatically picks out, or sometimes the machine can even cut through clutter that your brain won't. |
Winning the Lottery: Just what is the probability of winning the lottery? This short page will show you how to calculate your chances, and justify the lottery's alias: A Tax on People That Can't Do Math.
Fibonacci series: The first recursive series known to Europe. This article shows what the Fibonacci numbers are and how the recursive series works. It only hints at some of the amazing applications of the series.
The Golden Ratio, a fascinating number. The Golden Ratio has applications to aesthetics, biology and other sciences, this article shows one example.
The quadratic formula is a quick, easily recalled formula for solving a quadratic equation. Quadratic equations show up in all kinds of science and engineering problems as well as your high school homework. This short article shows how to derive the quadratic formula just in case you forgot the formula or you are just going crazy trying to remember how you derived it once a long time ago.
A Taylor series expansion can turn a complicated function into a simple power series polynomial, sometimes. This little trick is very useful in science and engineering and especially in computer modeling of physical phenomena when working with functions that are difficult and time consuming to calculate values for.
Finding the angle of a 2 dimensional distribution Continuous or discrete 2D distributions have a best fit angle. Here is how to find it.
Zernike Polynomials used for representation of light beam wavefronts. Represent any 2-D surface as long as it has a circular footprint.
Zernike Polynomials MathCAD sheet, in mathcad 2001i format. . This sheet calculates the zernike polynomial of your choice and displays a 3D graph. (Requires mathcad from mathsoft)
 Get this wonderful home study course on human anatomy in 3-D on your computer before the price goes up!. |
$18M in Affiliate Secrets!